Solid Dynamics: Geometrically Exact Beam Finite Elements
Christoph Meier, Alexander Popp and Wolfgang A. Wall
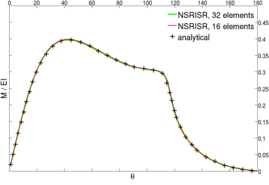
A beam formulation is denoted as geometrically exact when the relationships between the configuration and the strain measures are consistent with the virtual work principle and the equilibrium equations at a deformed state regardless of the magnitude of displacements, rotations and strains. This category of beam formulations is typically characterized by both, high accuracy and high efficiency. While most of the geometrically exact beam formulations available in the literature are of Simo-Reissner type, thus incorporating the modes of torsion, bending, axial tension and shear, our research work concentrates on the development of geometrically exact Kirchhoff formulations which are tailored to deal with very high slenderness ratios and related numerical challenges such as different locking phenomena, ill-conditioning or time integration instabilities.
The neglect of shear deformation and the related high stiffness contributions, which is the essential difference of these elements as compared to beam elements of Simo-Reissner type, is not only a valid assumption in the regime of high slenderness ratios, it also provide some potential advantages concerning the above mentioned challenges. This path can be trodden even further by additionally assuming the beam centerline as being inextensible and thus neglecting also the second high stiffness contribution, the axial tension. Moreover, in many fields of application, some modes of deformation (e.g. torsion) can be neglected within thin fibers. This is our motivation for the development of torsion-free beam elements, a category of formulations that can completely abstain from the use of rotational degrees of freedom and all complexities related with the latter.