Multiscale Problems
The interplay of effects on different scales plays an important role in many scientific and engineering applications. Therefore, there has been increasing interest in modeling so-called multiscale phenomena both mathematically and computationally.
Turbulent flows, for example, are characterized by a wide range of length and time scales. Our methodical approach for solving this kind of flow problems is based on multiscale and stabilized methods. Important applications include chemically-reacting flows, electrodeposition of metals and combustion.
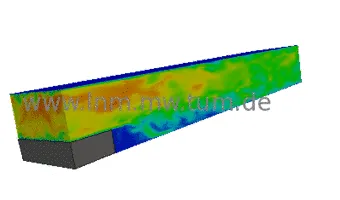
Our research also focuses on the modeling of heterogeneous materials. To enable the effective analysis of these materials, we developed a novel multiscale (FE2) method for 3D nonlinear solid dynamics. One application of this approach is the modeling of lung tissue dynamics on different length scales. In combination with optimization methods, FE2 methods can also be used to deduce micro-level material properties from macroscopic experiments in terms of a multiscale inverse analysis.